Correlation of powder flow properties to interparticle interactions at ambient and high temperatures--颗粒学报PARTICUOLOGY

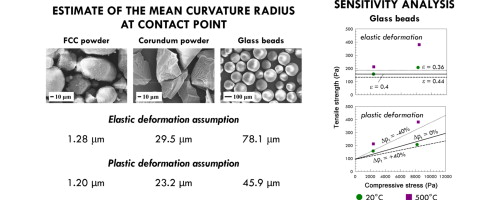
Tomasetta, I., Barletta, D., & Poletto, M. (2014). Correlation of powder flow properties to interparticle interactions at ambient and high temperatures. Particuology, 12, 90–99. https://doi.org/10.1016/j.partic.2013.02.002

Correlation of powder flow properties to interparticle interactions at ambient and high temperatures

Igino Tomasetta, Diego Barletta, Massimo Poletto^*

Dipartimento di Ingegneria Industriale, Università degli Studi di Salerno, 84084 Fisciano, SA, Italy

10.1016/j.partic.2013.02.002

Volume 12, February 2014, Pages 90-99

Received 30 November 2012, Revised 31 January 2013, Accepted 28 February 2013, Available online 26 April 2013.

E-mail: mpoletto@unisa.it; poletto.massimo@gmail.com

Highlights

• Powder tensile strength is related to van der Waals interparticle forces.

• Rumpf equation is adopted.

• Elastic and plastic deformation at contact points is alternatively assumed.

• Plastic deformation and voidage decrease describe the effect of consolidation.

• Changes of the material strength can explain the effect of temperature.

Abstract

A combination of a continuum approach and a particle–particle approach to describe the multi-scale nature of the mechanical properties of bulk solids may be beneficial to scientific and engineering applications. In this paper, a procedure is proposed to estimate the interparticle forces beginning with the bulk flow properties as measured with standardized techniques. In particular, the relationship between interparticle forces and bulk solid tensile strength is adopted based on the microscale approaches of Rumpf (1970) and Molerus (1975). The flow properties of fluid cracking catalyst (FCC), corundum and glass bead powders were all characterized with a modified Schulze ring shear cell capable of operating at temperatures up to 500 °C. The powder test conditions were selected such that the van der Waals forces were the most significant particle–particle interactions. The model equations describe two cases, in which either elastic or plastic deformation of the contact points is assumed. The results indicate that the model provides the correct order of magnitude for the values of the tensile strength when proper values for the mean curvature radius at the contact points are taken into account. A sensitivity analysis for the main parameters in the model was performed. This analysis indicated that the assumption of plastic deformation at contact surfaces coupled with a decrease in porosity justified an increase of the tensile strength with consolidation stress. Furthermore, the effect of temperature on the measured flow behavior can be explained as a change in the strength of the material.

Graphical abstract

Keywords

Shear testing; Yield locus; van der Waals force; Interparticle interactions; Powder consolidation; High temperature

文章导读