Revisiting rolling and sliding in two-dimensional discrete element models--颗粒学报PARTICUOLOGY

Wang, Y., Alonso-Marroquin, F., Xue, S., & Xie, J. (2015). Revisiting rolling and sliding in two-dimensional discrete element models. Particuology, 18, 35–41. https://doi.org/10.1016/j.partic.2014.04.013

Revisiting rolling and sliding in two-dimensional discrete element models

Yucang Wang^{a b *}, Fernando Alonso-Marroquin ^c, Sheng Xue ^b, Jun Xie ^b

a School of Engineering and Technology, Central Queensland University, Australia
b Earth Science and Resource Engineering, The Commonwealth Scientific and Industrial Research Organisation (CSIRO), Australia
c School of Civil Engineering, The University of Sydney, Australia

10.1016/j.partic.2014.04.013

Volume 18, February 2015, Pages 35-41

Received 3 March 2014, Revised 15 April 2014, Accepted 16 April 2014, Available online 18 August 2014.

E-mail: y.wang2@cqu.edu.au; yucang_wang@hotmail.com

Highlights

• Some previous research work overlooked the effect of particle size on particle rolling and sliding.

• Clear definitions for pure rolling and pure sliding were made for two particles of different sizes.

• A unique solution of rolling velocity was reached, leading to consistencies between different models.

Abstract

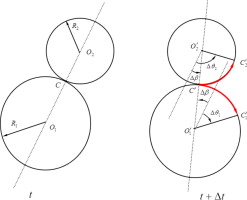
It has long been recognized that the rotation of single particles plays a very important role in simulations of granular flow using the discrete element method (DEM). Many researchers have also pointed out that the effect of rolling resistance at the contact points should be taken into account in DEM simulations. However, even for the simplest case involving two-dimensional circular particles, there is no agreement on the best way to define rolling and sliding, and different definitions and calculations of rolling and sliding have been proposed. It has even been suggested that a unique rolling and sliding definition is not possible. In this paper we assess results from previous studies on rolling and sliding in discrete element models and find that some researchers have overlooked the effect of particles of different sizes. After considering the particle radius in the derivation of rolling velocity, all results reach the same outcome: a unique solution. We also present a clear and simple derivation and validate our result using cases of rolling. Such a decomposition of relative motion is objective, or independent of the reference frame in which the relative motion is measured.

Graphical abstract

Keywords

Discrete element method; Rolling; Sliding

文章导读