Disordered packing density of binary and polydisperse mixtures of curved spherocylinders--颗粒学报PARTICUOLOGY

Meng, L., & Li, S. (2017). Disordered packing density of binary and polydisperse mixtures of curved spherocylinders. Particuology, 32, 73-81. https://doi.org/10.1016/j.partic.2016.05.018

Disordered packing density of binary and polydisperse mixtures of curved spherocylinders

Lingyi Meng ^a *, Shuixiang Li ^b *

a Department of Mechanics Engineering, School of Civil Engineering and Transportation, South China University of Technology, Guangzhou 510641, China
b Department of Mechanics & Aerospace Engineering, College of Engineering, Peking University, Beijing 100871, China

10.1016/j.partic.2016.05.018

Volume 32, June 2017, Pages 73-81

Received 16 March 2016, Revised 10 May 2016, Accepted 26 May 2016, Available online 4 January 2017, Version of Record 20 April 2017.

E-mail: ctlymeng@scut.edu.cn; lsx@pku.edu.cn

Highlights

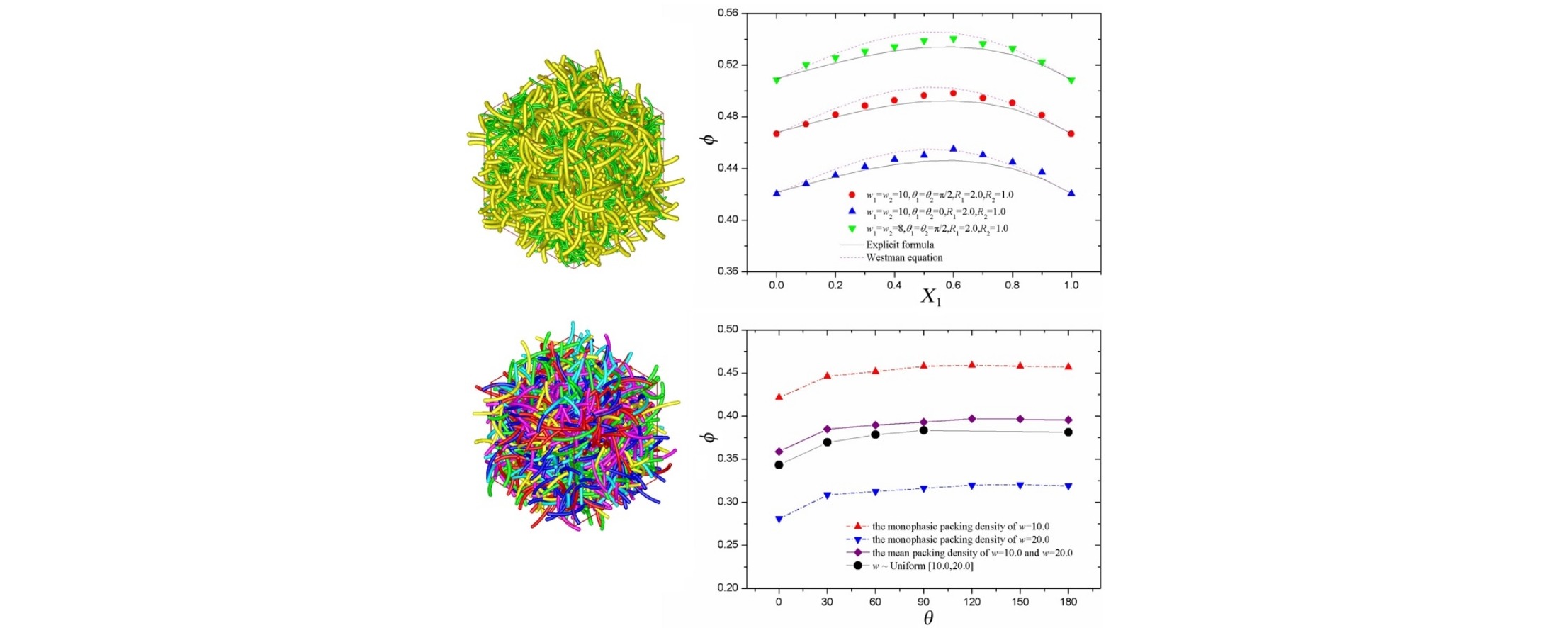
• Binary and polydisperse mixtures of curved spherocylinders were simulated via sphere assembly models and relaxation algorithm.

• Shape and size independently influenced the mixture density of binary curved spherocylinders.

• The explicit formula was extended to include a non-convex shape factor.

• Packing density of polydisperse mixture was equivalent to binary mixture with certain components.

Abstract

Particle elongation is an important factor affecting the packing properties of rod-like particles. However, rod-like particles can be easily bent into non-convex shapes, in which the effect of bending should also be of concerned. To explore the shape effects of elongation and bending, together with the size and volume fraction effects on the disordered packing density of mixtures of non-convex particles, binary and polydisperse mixtures of curved spherocylinders are simulated employing sphere assembly models and the relaxation algorithm in the present work. For binary packings with the same volume, curves of the packing density versus volume fraction have good linearity, while densities are plotted as a series of equidistant curves under the condition of the same shape. The independence of size and shape effects on the packing density is verified for mixtures of curved spherocylinders. The explicit formula used to predict the density of binary mixtures, by superposing the two independent functions of the size and shape parameters, is extended to include a non-convex shape factor. A polydisperse packing with the shape factor following a uniform distribution under the condition of the same volume is equivalent to a binary mixture with certain components. The packing density is thus predicted as the mean of maximum and minimum densities employing a weighing method.

Graphical abstract

Keywords

Disordered packing; Packing density; Mixture; Curved spherocylinder; Non-convex particle

文章导读