Numerical simulation of fluidized dense-phase pneumatic conveying of powders to develop improved model for solids friction factor--颗粒学报PARTICUOLOGY

Kaur, B., Mittal, A., Mallick, S. S., Pan, R., & Jana, S. (2017). Numerical simulation of fluidized dense-phase pneumatic conveying of powders to develop improved model for solids friction factor. Particuology, 35, 42-50. https://doi.org/10.1016/j.partic.2016.11.006

Numerical simulation of fluidized dense-phase pneumatic conveying of powders to develop improved model for solids friction factor

Baldeep Kaur ^a, Anu Mittal ^b *, S.S. Mallick ^b, Renhu Pan ^c, Soumendu Jana ^a

a School of Physics and Materials Science, Thapar University, Patiala, Punjab 147004, India
b Department of Mechanical Engineering, Thapar University, Patiala, Punjab 147004, India
c Fujian Longking Co., Ltd., Longyan, Fujian 364000, China

10.1016/j.partic.2016.11.006

Volume 35, December 2017, Pages 42-50

Received 16 June 2016, Revised 14 November 2016, Accepted 22 November 2016, Available online 9 May 2017, Version of Record 30 November 2017.

E-mail: anu.mittal@thapar.edu

Highlights

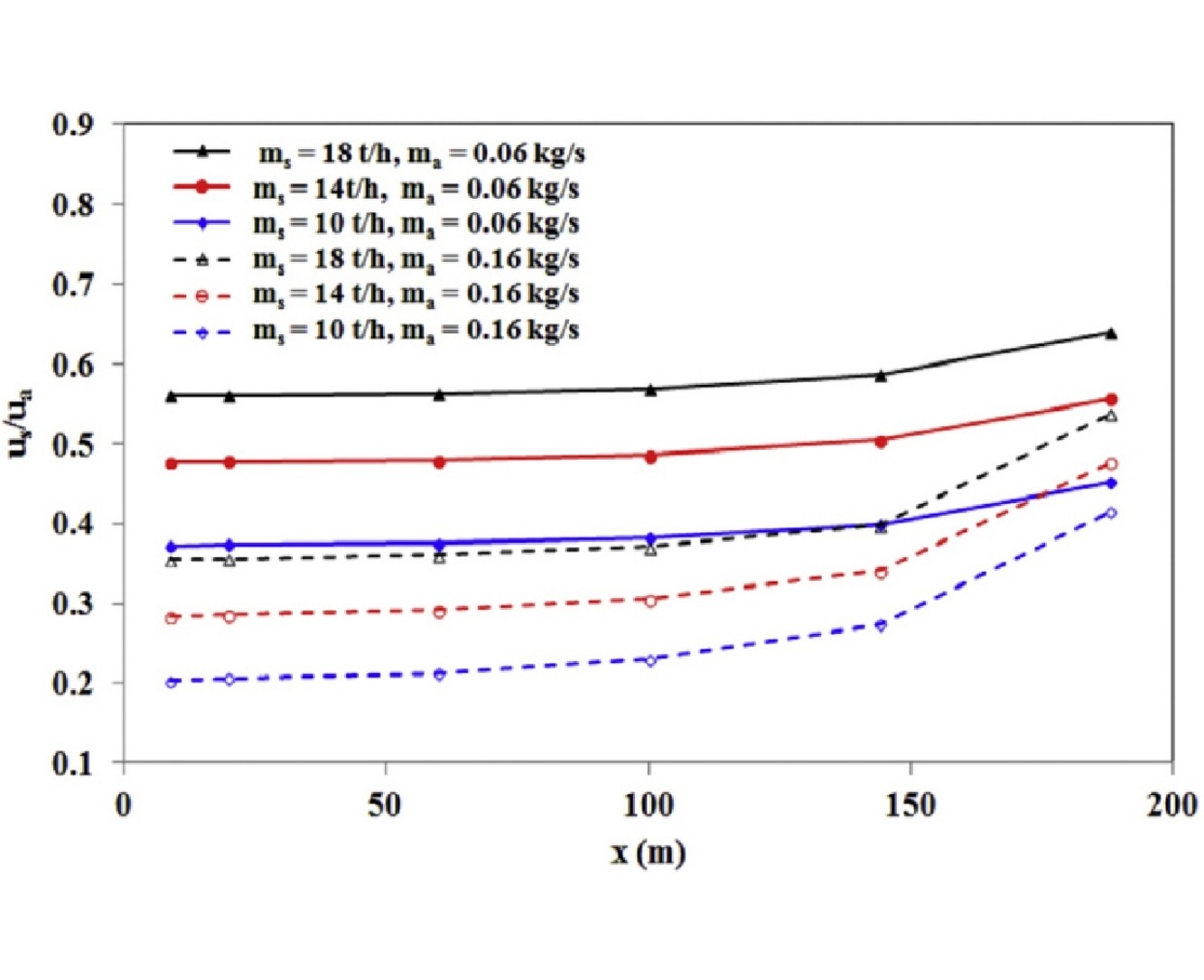
• Numerical simulations showed increased particle and actual air velocities along flow direction.

• New model for solids friction factor was developed using particle-to-air velocity ratio.

• New model was evaluated for scale-up accuracy in longer and larger pipeline.

Abstract

Accurate prediction of the solids friction factor through horizontal straight pipes is important for the reliable design of a pneumatic conveying system, but it is a challenging assignment to date because of the highly concentrated, turbulent, and complex nature of the gas–solids mixture. Power-station fly ash was transported through different pipeline configurations. Numerical simulation of the dense-phase pneumatic conveying systems for three different solids and two different air flow rates have shown that particle and actual gas velocities and the ratio of the two velocities increases in the flow direction, whereas the reverse trend was found to occur for the solids volumetric concentration. To develop a solids friction-factor model suitable for dense-phase flow, we modified an existing pure dilute-phase model by incorporating sub-models for particle and actual gas velocities and impact and solids friction factor. The solids friction-factor model was validated by using it for scale-up predictions for total pipeline pressure drops in longer and larger pipes and by comparing experimental and predicted pneumatic conveying characteristics for different solids flow rates. The accuracy of the prediction was compared with a recently developed two-layer-based model. We discussed the effect of incorporating the particle and actual gas velocity terms in the solids friction-factor model instead of superficial air velocity.

Graphical abstract

Keywords

Pneumatic conveying; Dense-phase; Numerical analysis; Pressure drop; Scale-up validation

文章导读