Discrete element method dynamic simulation of icosahedral particle packing under three-dimensional mechanical vibration--颗粒学报PARTICUOLOGY

Zhang, G., An, X., Zhao, B., Qian, Q., & Zhao, H. (2019). Discrete element method dynamic simulation of icosahedral particle packing under three-dimensional mechanical vibration. Particuology, 44, 117-125. https://doi.org/10.1016/j.partic.2018.03.004

Discrete element method dynamic simulation of icosahedral particle packing under three-dimensional mechanical vibration

Guangjian Zhang ^a, Xizhong An ^a *, Bo Zhao ^{a b}, Quan Qian ^a, Haiyang Zhao ^a

a School of Metallurgy, Northeastern University, Shenyang 110004, China
b School of Materials Science and Engineering, Northeastern University, Shenyang 110004, China

10.1016/j.partic.2018.03.004

Volume 44, June 2019, Pages 117-125

Received 17 January 2018, Revised 9 February 2018, Accepted 2 March 2018, Available online 25 July 2018, Version of Record 30 April 2019.

E-mail: anxz@mail.neu.edu.cn

Highlights

• Vibrated packings of monosized regular icosahedral particles were numerically modeled by DEM.

• Transformation from random loose packing to random close packing was reproduced.

• Influences of operating parameters on the packing densification were identified and optimized.

• Various macro and micro properties of the packing structures were characterized and compared.

Abstract

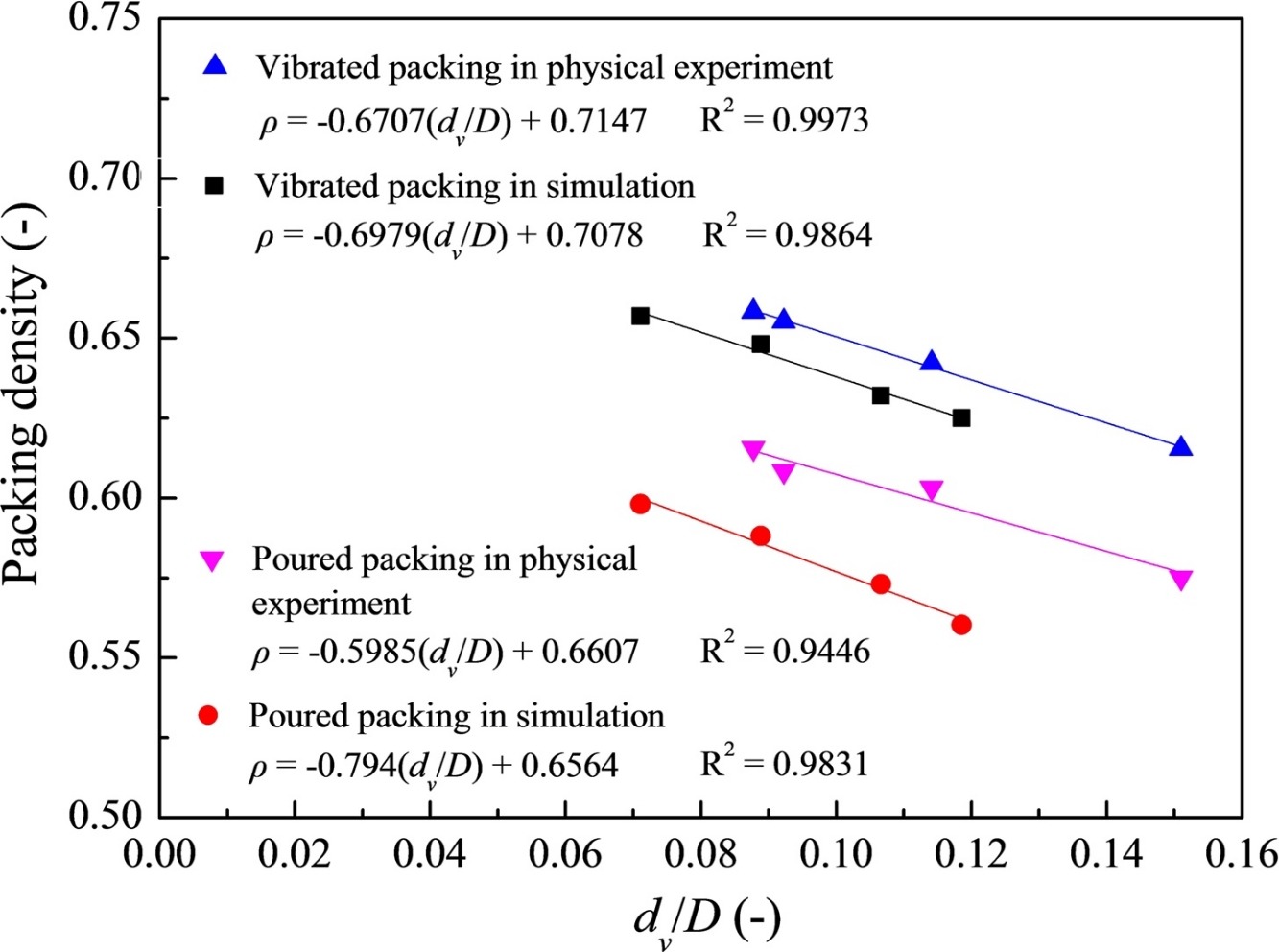
Packing densification of monosized regular icosahedral particles under three-dimensional mechanical vibration has been simulated by the discrete element method (DEM). The effects of the vibration conditions and container size on packing densification were systematically investigated. In addition to the macroscale properties (packing density and porosity), the microscale properties, such as the coordination number (CN), radial distribution function (RDF), particle contact type, particle orientation distribution, and stresses/forces, in random loose packing (RLP) and random close packing (RCP) were also characterized and analyzed. The results show that transformation of icosahedral particle packing from RLP to RCP can be realized by properly controlling the vibration conditions. The maximum random packing density without the wall effect reaches 0.7078. Microscale property analysis shows that the average CN increases after vibration. The RDF curves contain two clear peaks for RLP and three for RCP. From RLP to RCP, the probability of face to face contact between two particles increases, while the probabilities of edge to edge, edge to face, and face to vertex contact decrease. The orientation correlation functions indicate the randomness of the vibrated packing structure. In addition, more uniform force and stress distributions are observed within the dense packing structure.

Graphical abstract

Keywords

Icosahedral particles; Packing densification; Mechanical vibration; Discrete element method simulation

文章导读