Wavelet denoising and nonlinear analysis of solids concentration signal in circulating fluidized bed riser--颗粒学报PARTICUOLOGY

Gu, L.-L., Zhang, Y., & Zhu, J. (2020). Wavelet denoising and nonlinear analysis of solids concentration signal in circulating fluidized bed riser. Particuology, 49, 105-116. https://doi.org/10.1016/j.partic.2019.01.005

Wavelet denoising and nonlinear analysis of solids concentration signal in circulating fluidized bed riser

Li-Li Gu ¹, Yawen Zhang ^*, Jesse Zhu

Received 2 April 2018, Revised 8 October 2018, Accepted 8 January 2019, Available online 2 July 2019, Version of Record 26 February 2020.

10.1016/j.partic.2019.01.005

Volume 49, April 2020, Pages 105-116

Received 2 April 2018, Revised 8 October 2018, Accepted 8 January 2019, Available online 2 July 2019, Version of Record 26 February 2020.

E-mail: ezhang26@uwo.ca; zhangyw@tju.edu.cn

Highlights

• An efficient denoising algorithm is applied to dynamic signals in a CFB via the wavelet domain.

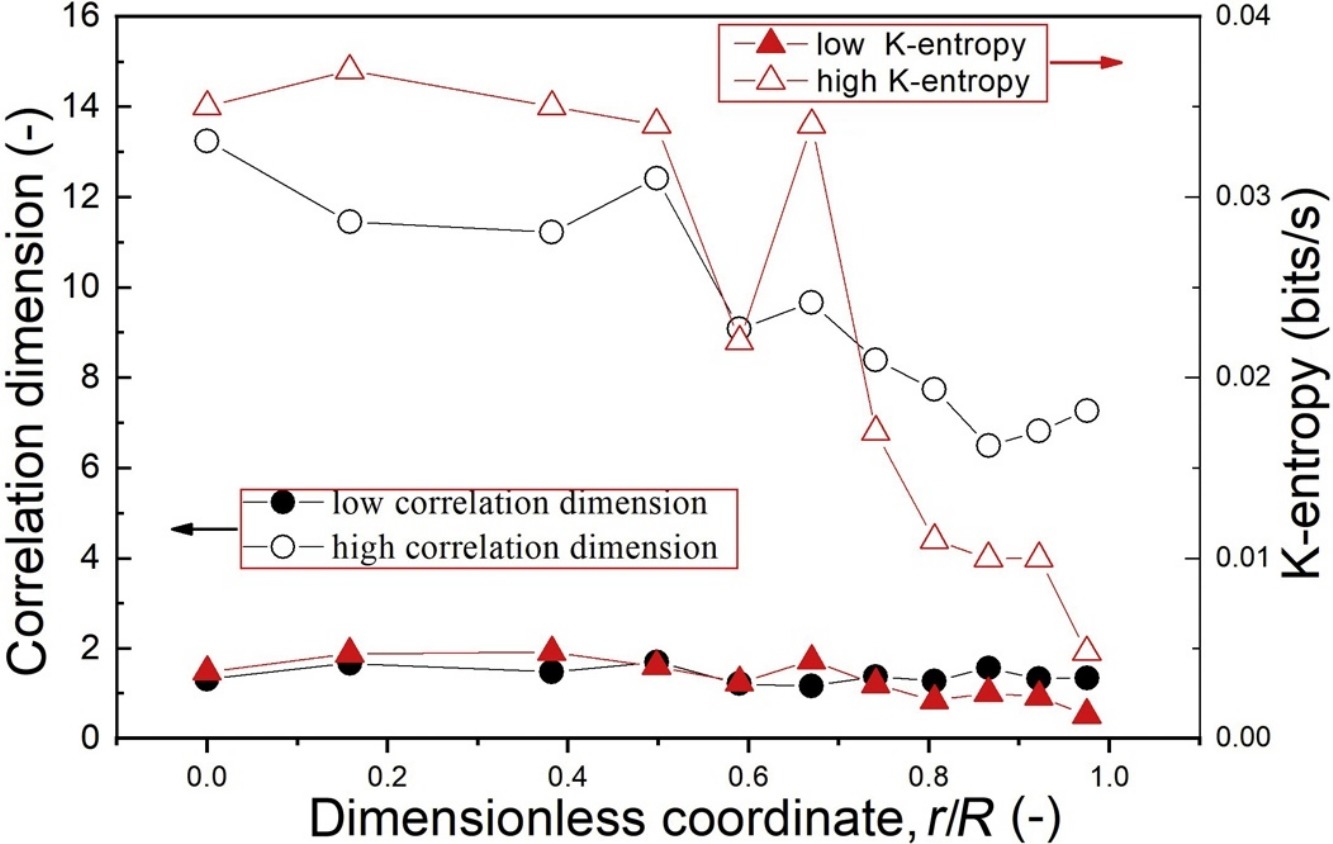
• The correlation dimension and Kolmogorov entropy were calculated using denoised data.

• First (lower) and second (higher) correlation dimensions coexist in low-flux CFBs.

• The particle behavior has higher degrees of freedom at the center.

Abstract

Particles, particle aggregates, and reactor walls complicate the dynamic microstructures of circulating fluidized beds (CFBs). Using local solids concentration data from a 10-m-high and 76.2-mm-inner-diameter riser with FCC (Fluid Catalytic Cracking) particles (d_p = 67 μm, ρ_p = 1500 kg/m³), this paper presents an improved denoising process for use before nonlinear chaos analysis. Using the soft-threshold denoising method in the wavelet domain with experimental empty bed signals as base data to estimate the denoising threshold, an efficient denoising algorithm was proposed and used for the dynamic signals in CFBs. Analysis shows that for the local solids concentration time series, high-frequency fluctuations may be one of the system properties, while noise interference can also make a low-frequency contribution. An exact denoising method is needed in such cases. The correlation dimension and Kolmogorov entropy were calculated using denoised data and the results showed that the particle behavior in the CFB is highly complex. Generally, two correlation dimensions coexist in a low-flux CFB. The first correlation dimension is low and corresponds to small-scale fluctuations that reveal a high-frequency pseudo-periodic movement, but the second correlation dimension is high and corresponds to large-scale fluctuations that indicate multi-frequency movements, including particle aggregation and breakage. At the same axial level, the first correlation dimensions change slightly with radial position, and the main tendency is high at the center but slightly lower near the wall. However, the second correlation dimensions show large changes along the radial direction, are again high in the core region, and after r/R ≥ 0.6 (r as radial position, R as radius of the riser), the dimensions clearly drop down. This indicates that the particle behavior is more complex and has higher degrees of freedom at the center, but clusters near the wall are restrained to some degree because of wall effects.

Graphical abstract

Keywords

Circulating fluidized bed riser; Wavelet transform; Soft threshold denoising; Time delay embedding; Correlation dimension; Kolmogorov entropy

文章导读