Complex granular flows of sticky-wet material on flip-flow screens: Calibration of discrete element simulations--颗粒学报PARTICUOLOGY

Xu, N., Tang, S., Lin, D., Geng, R., Wang, X., & Liang, X. (2024). Complex granular flows of sticky-wet material on flip-flow screens: Calibration of discrete element simulations. Particuology, 84, 290-308. https://doi.org/https://doi.org/10.1016/j.partic.2023.07.010

Complex granular flows of sticky-wet material on flip-flow screens: Calibration of discrete element simulations

Ningning Xu^{a b}, Sen Tang^a, Dongdong Lin^a, Runhui Geng^a, Xinwen Wang^a *, Xihui Liang^b

a School of Chemical and Environmental Engineering, China University of Mining and Technology (Beijing), Beijing, 100083, China
b Department of Mechanical Engineering, University of Manitoba, Winnipeg, Manitoba, R3T 5V6, Canada

10.1016/j.partic.2023.07.010

Volume 84, January 2024, Pages 290-308

Received 29 March 2023, Revised 13 June 2023, Accepted 14 July 2023, Available online 28 July 2023, Version of Record 1 August 2023.

E-mail: xinwen.w@cumtb.edu.cn

Highlights

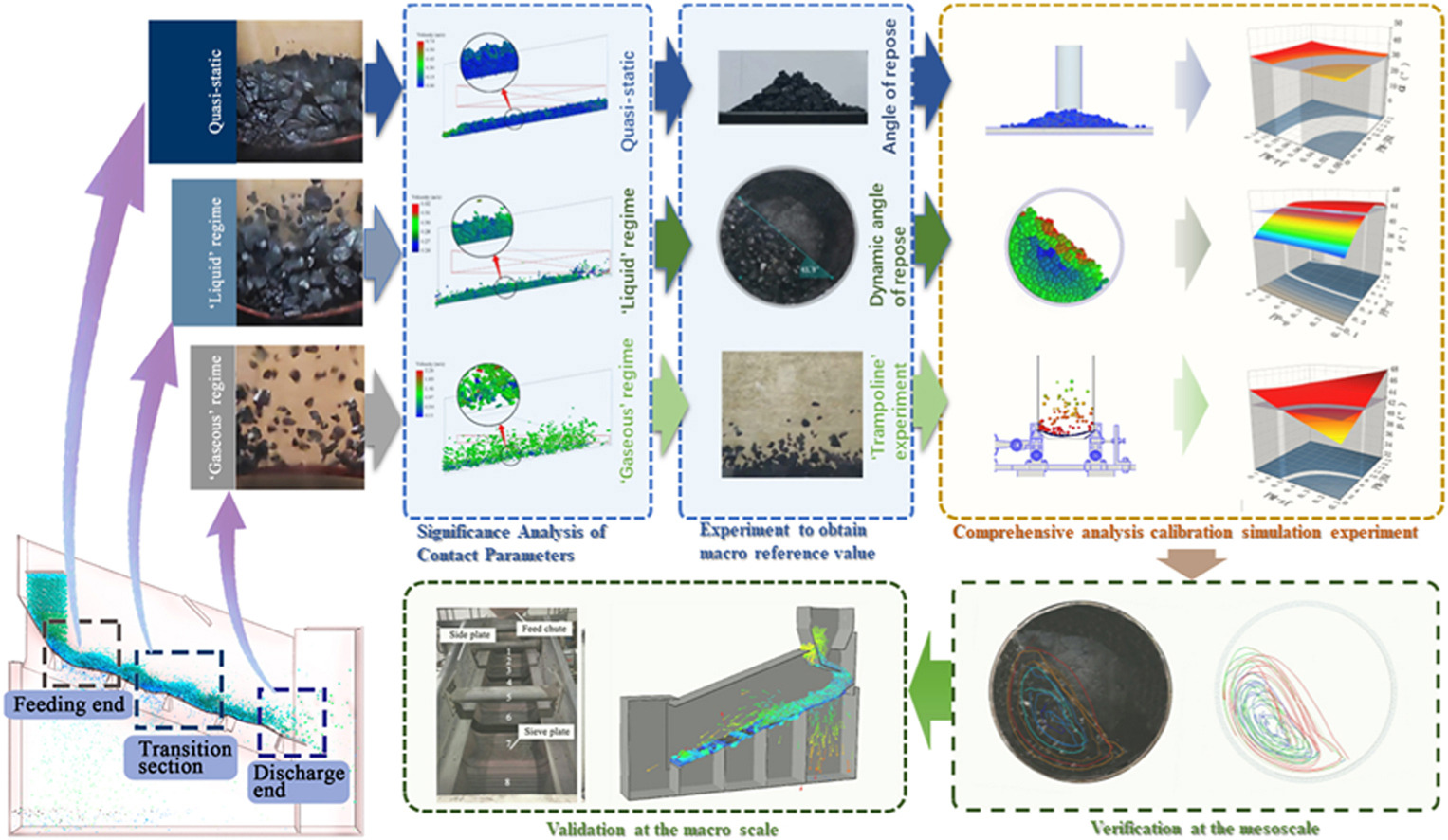
• A multi-regime combination calibration method based on particle flow regime characteristics was proposed.

• Dominant contact parameters for different flow regimes on a vibrating bed were studied.

• Calibration process was validated on both macroscale and mesoscale.

• A trampoline test was designed to calibrate the contact parameters in “gaseous” regime flow.

Abstract

Discrete element method (DEM) is an effective approach for studying the screening process of flip-flow screens. However, there have been few studies focusing on the thick layer of sticky-wet particles on flip-flow screens. To achieve accurate simulations of the thick layer of sticky-wet particles on a flip-flow screen, firstly, the movement law of particle flow was studied, and a multi-regime combination calibration method based on characteristics of particle flow regimes was proposed. Based on the Plackett-Burman experiment, the curse of dimensionality caused by multi-state and multi-contact parameters was overcome. Subsequently, the lifting cylinder, rotating drum, and trampoline tests were carried out to obtain macroscopic reference values under various granular flow regimes. The calibration results were then determined using the response surface method and climbing algorithm. Finally, the calibration results were tested at both macroscopic and mesoscopic scales and compared with a commonly used calibration method. The results demonstrate that the calibration method, which considers the multi-state characteristics, improves simulation accuracy by 2%–10% and reduces the simulation error to less than 10%, thus meeting the requirements for engineering optimization of flip-flow screens.

Graphical abstract

Keywords

DEM; Complex granular flow; Parameter calibration; Flip-flow screen; Sticky-wet particles; Thick layer

文章导读