Numerical simulations of gas-liquid-solid circulating flows coupled to mesoscale parameters and measurements--颗粒学报PARTICUOLOGY

Zhang, L., Ma, Y., & Liu, M. (2025). Numerical simulations of gas-liquid-solid circulating flows coupled to mesoscale parameters and measurements. Particuology, 97, 236-256. https://doi.org/10.1016/j.partic.2024.12.013

Numerical simulations of gas-liquid-solid circulating flows coupled to mesoscale parameters and measurements

Lubin Zhang^a, Yongli Ma^a *, Mingyan Liu ^{a b}

a School of Chemical Engineering and Technology, Tianjin University, Tianjin, 300350, China
b State Key Laboratory of Chemical Engineering, Tianjin, 300350, China

10.1016/j.partic.2024.12.013

Volume 97, February 2025, Pages 236-256

Received 18 September 2024, Revised 16 December 2024, Accepted 19 December 2024, Available online 13 January 2025, Version of Record 20 January 2025.

E-mail: mayl@tju.edu.cn

Highlights

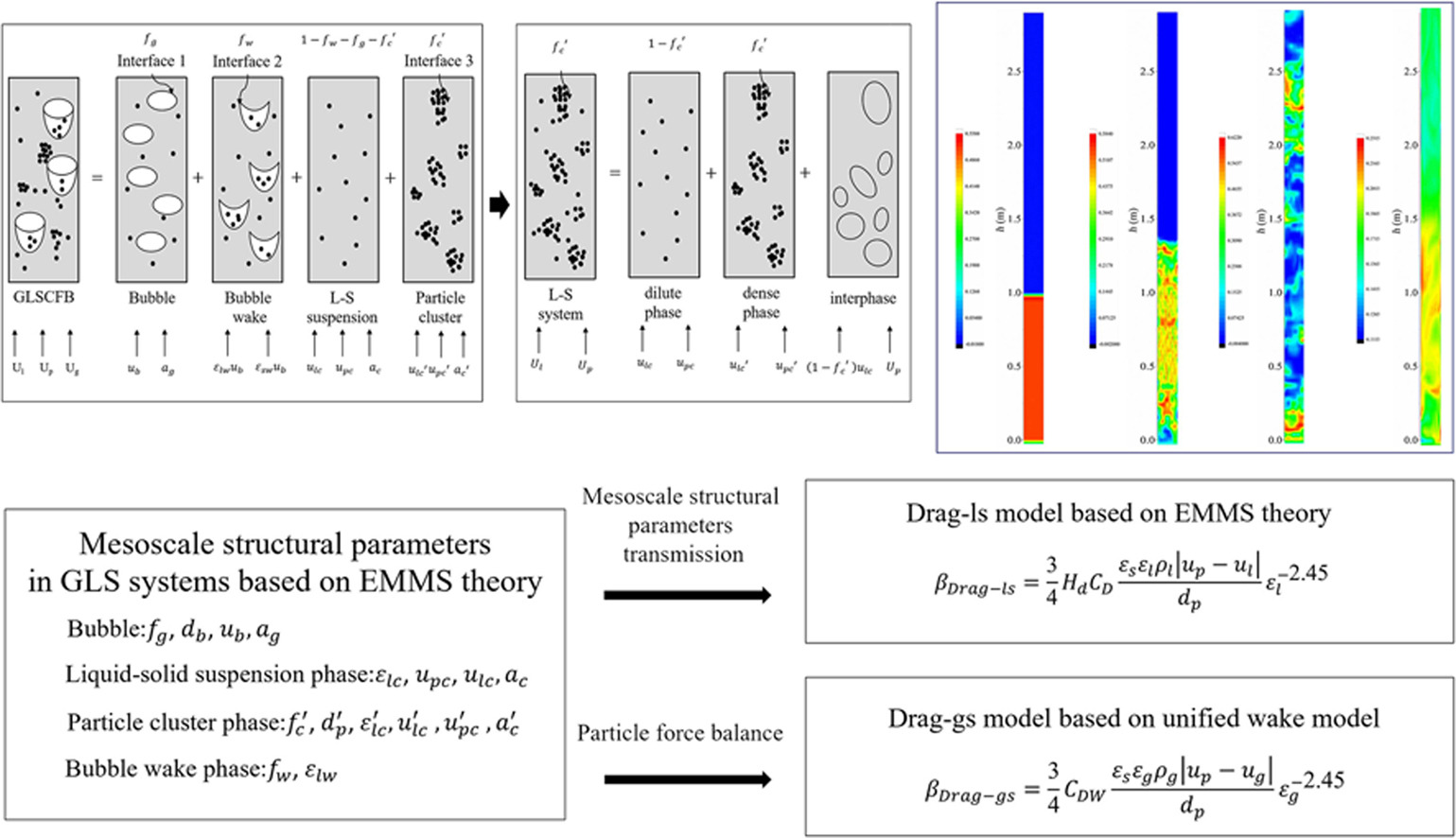
• Liquid-solid drag coefficient in GLSCFB is higher than that in LSCFB.

• Unified wake model has been modified by mesoscale parameters based on EMMS theory.

• Phase holdup is more accurately simulated by combining EMMS theory and CFD method.

Abstract

Gas-liquid-solid circulating fluidized bed (GLSCFB) is an important type of chemical reactor. The complex mesoscale flow structure of GLSCFB was described through the mesoscale flow structure parameters based on the energy-minimization multi-scale (EMMS) model. The liquid-solid drag force model (Drag-ls model) was proposed and it was found that the drag coefficient between liquid and solid in three-phase systems increased compared to liquid-solid two-phase systems because of the influence of gas phase. The gas-solid drag force model (Drag-gs model) was proposed based on a modified unified wake model. Furthermore, the combination of EMMS model and computational fluid dynamics (CFD) in GLSCFB was implemented, and the dynamic evolution process of particle clusters and distributions of gas holdup and solid holdup in GLSCFB were simulated more accurately by the models. The simulation results indicate that the drag forces exerted on the solid phase by both the liquid and gas phases are coupled and mutually influence each other. The simulated values of solid holdup may deviate from the experimental values if the interactions between the gas-solid and liquid-solid phases are corrected independently. When the average solid holdup of the bed is low, the mesoscale phenomena such as particle aggregation are not obvious. As the solid holdup increases, there is a significant phenomenon of particle aggregation in the bed. The particles undergo a spatiotemporal evolution process of forming elongated clusters with high solid holdup, spherical clusters with high solid holdup, and clusters with low solid holdup which has large surface areas.

Graphical abstract

Keywords

Gas-liquid-solid circulating fluidized bed; Energy-minimization multi-scale model; Unified wake model; Computational fluid dynamics; Particle cluster

文章导读